Best Hydraulic Cross- Section

Best Hydraulic Cross- Section

We often want to know the the minimum area A for a given flow Q, slope S₀ and roughness coef- ficient n.

This is known as the best hydraulic cross section

The quantity AR_h^2/3 in Mannings' equation is called the section factor

Writing the Manning equation with R_h = A/P, we get

· ( inside ) is a constant;  Channel with minimum A is also minimum P

· Minimum excavation area A also has minimum P

· Best possible is semicircular channel, but construction costs are high

Let's find out what the best hydraulic cross section is for a rectan- gular channel

Example: Water flows uniformly in a rectangular channel of width b and depth y. Determine the aspect ratio b/y for the best hydraulic cross section.

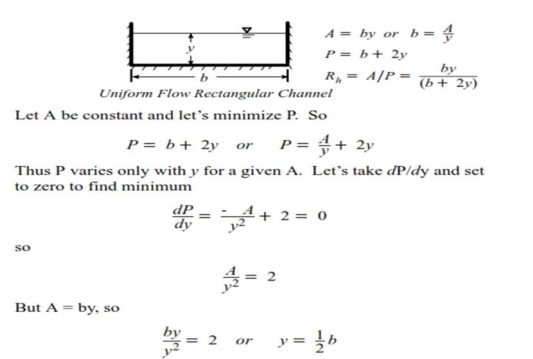
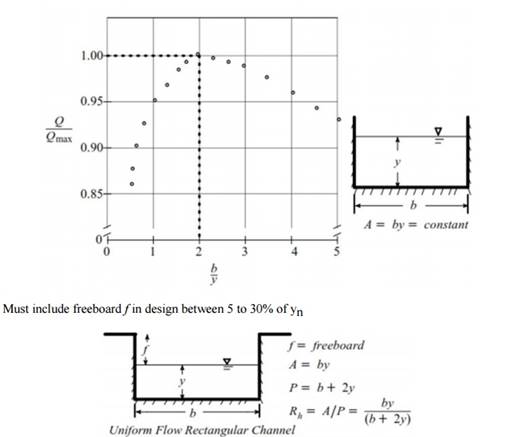
o Thus best hydraulic cross- section for a rectangular channel occurs when the depth is one-half the width of the channel

· Note for 1 < b/y < 4; Q �  .96 Q_max

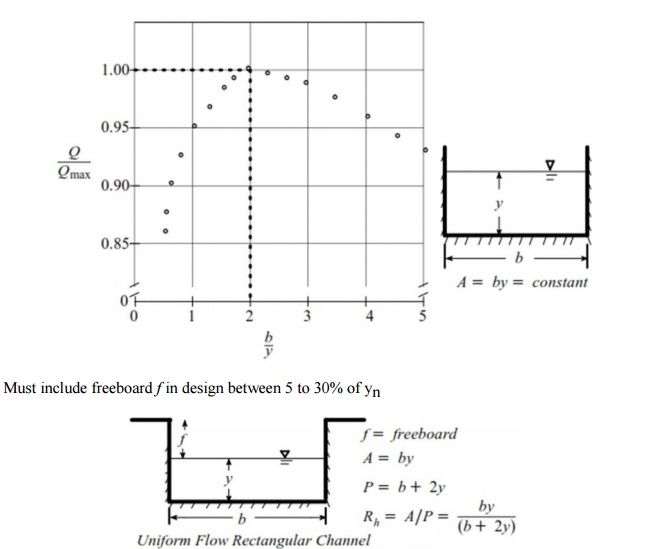
Must include freeboard f in design between 5 to 30% of y_n

Table gives Optimum properties of Open Channel Sections

.For trapezoid, half- hexagon

.For circular section, half- circle

.For triangular section, half- square

Design of Erodible Channels

Design velocity V small enough not to cause erosion

Find maximum permissible velocity based on channel material (Roberson, Table 4- 3)

Maximum Permissible Velocities a  nd n Values for Different       Materials   

Material     V(ft/s)                   n

Fine Sand   1.50            0.020

Sandy loam 1.75            0.020

Silt loam     2.00            0.020

Firm loam   2.50            0.020

Stiff clay     3.75            0.025

Fine gravel  2.50            0.025

Coarse gravel       4.00            0.025

Assuming a trapezoidal channel, maximum side slopes depend on material (Roberson,Table 4-2)

Maximum Channel Wall Slopes for Different Materials

Material : Side Slopes

Rock : Almost Vertical

Stiff clay or earth with concrete : 1/2 : 1 to 1:1

Firm Soil    1:1

Loose sandy soil  2:1

Sandy loam soil   3:1

Once  Q, V, n, S₀ are determined, solve for depth y and width b.

Problem: For an unlined trapezoidal irrigation canal in firm loam soil, slope is 0.0006 and flow is 100 cfs, what dimensions?

For side slope, pick slope of 1 1/2 (h): 1 (v) (conservative) V_max = 2.5 ft/s, n = 0.020

To find R_h

To construct choose b = 18 ft and y = 2.0 ft.

Critical Slope

o Holding n and Q constant, changing slope slope will change depth and velocity

o Where velocity and depth give a Froude number =1, this is defined as the critical slope S_c and crit- ical depth y_c