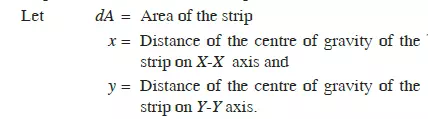Moment of Inertia

INTRODUCTION

We have already discussed in Art. 3.2 that the moment of a force (P) about a point, is the product of the force and perpendicular distance (x) between the point and the line of action of the force (i.e. P.x). This moment is also called first moment of force. If  this moment is again multiplied by the perpendicular distance (x) between the point and the line of action of the force i.e. P.x (x) = Px2, then this quantity is called moment of the moment of a force or second moment of force or moment of inertia (briefly written as M.I.). Sometimes, instead of force, area or mass of a

figure or body is taken into consideration. Then the second moment is known as second moment of area or second moment of mass. But all such second moments are broadly termed as moment of inertia. In this chapter, we shall discuss the moment of inertia of plane areas only.

MOMENT OF INERTIA OF A PLANE AREA

Consider a plane area, whose moment of inertia is required to be found out. Split up the whole area into a number of small elements.

UNITS OF MOMENT OF INERTIA

As a matter of fact the units of moment of inertia of a plane area depend upon the units of the area and the length. e.g.,

METHODS FOR MOMENT OF INERTIA

The moment of inertia of a plane area (or a body) may be found out by any one of the following two methods :

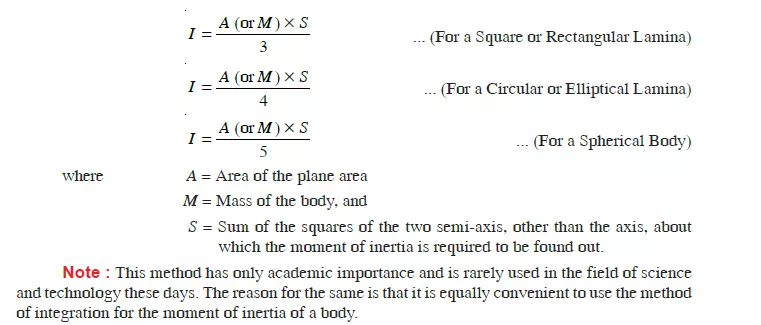
MOMENT OF INERTIA BY ROUTH’S RULE

The Routh’s Rule states, if a body is symmetrical about three mutually perpendicular axes*,

then the moment of inertia, about any one axis passing through its centre of gravity is given by:

MOMENT OF INERTIA BY INTEGRATION

The moment of inertia of an area may also be found out by the method of integration as discussed below:

Consider a plane figure, whose moment of inertia is required to be found out about X-X axis and Y-Y axis as shown in Fig 7.1. Let us divide the whole area into a no. of strips. Consider one of these strips.