Derivation of Unit Hydrographs
Derivation of Unit Hydrograph from a Simple Storm
A number of isolated storm hydrographs caused by short spells of rainfall excess each of approximately same duration are elected from a. study of the continuously gauged runoff of the stream. For each of these storm hydrograph, the base flow is separated. The area under each DRH is evaluated and the volume of the direct runoff obtained is divided by the catchment area to obtain the depth of ER. The ordinates of the various DRHs are divided by the respective ER values to obtain the ordinates of the Unit hydrograph.
Flood hydrograph used in the analysis should be selected to meet the following desirable features with respect to the storm responsible for them.
· The storms should be isolated storms occurring individually.
· The rainfall should be fairly uniform during the duration and should cover the entire catchment area.
· The duration of the rainfall should be 1/5 to 1/3of the basin lag.
· The rainfall excess of the selected storm should be high. A range of ER values of 1.0 to 4.0 cm is sometimes preferred.
Example 1
A flood hydrograph of a river draining a catchment of 189 km2 due to a 6 h isolated storm is in the form of a triangle with a base of 66 h and a peak ordinate of 30 m3/s occurring at 10 hours from the start. Assuming zero base flow, develop the 6-hour unit hydrograph for this catchment.
Answer
Given: catchment area (A) = 189 km2
Time interval = 6 h
Time base = 66 h
Peak = 30 m3/s
To find: 6-h UH for catchment
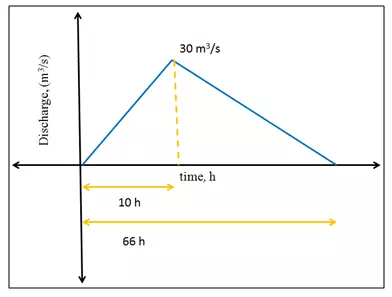
From figure
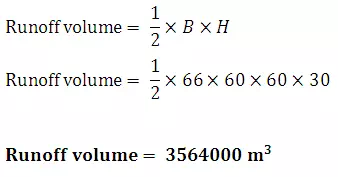
Therefore,
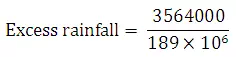
Excess Rainfall = 1.89 cm
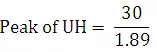
Peak of UH = 15.909 m2/s at 10 h from start
Derivation of Unit Hydrograph from a Complex Storm
When suitable simple isolated storm are not available, data from complex storms of long duration will have to be used in unit-hydrograph derivation.
Consider a rainfall excess made up of three consecutive durations of D-h and ER values of R1, R2, R3.Figure 25.1 shows the ERR By base flow separation of the resulting composite flood hydrograph a composite DRH is obtained (Fig. 25.1).
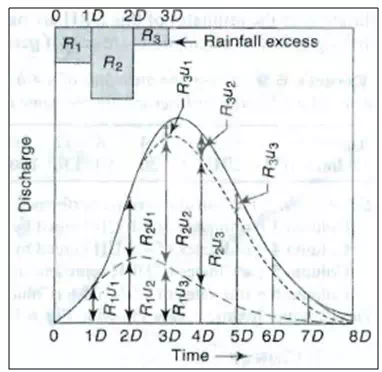
Fig..1.Unit hydrograph from a Complex storm.
Let the ordinates of composite DRH be drawn at a time interval of D-h. At various time intervals 1D, 2D, 3D,… from the start of ERH, let the ordinates of the unit hydrograph be u1, u2, u3,… and the ordinates of the composite DRH be Q1, Q2, Q3,…,
Then
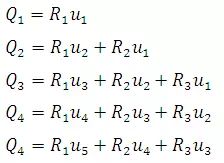
so on
From Eq..1 the values of u1, u2, u3…… can be determined.
However, this method suffers from the disadvantage that the errors propagate and increase as the calculations proceed. In the presence of errors the recession limb of the derived D-hunit hydrograph can contain oscillations and even negative values.
Example 1
The ordinates of the 6-h unit hydrograph of a basin are given:
|
Time |
h |
0 |
6 |
12 |
18 |
24 |
30 |
36 |
42 |
48 |
54 |
60 |
66 |
|
Ordinate of 6-h UH |
m3/s |
0 |
20 |
60 |
150 |
120 |
90 |
66 |
50 |
32 |
20 |
10 |
0 |
If two storms, each of 1-cm rainfall excess and 6-h duration occur in succession, calculate the resulting hydrograph of flow. Assume the base flow to be uniform at 10m3/s.
Answer
|
C1 |
C2 |
C3=C2*1 |
C4=C2*1 |
C5= C3+C4 |
C6 |
C7= C5+C6 |
|
Time |
Ordinate of |
DRH due to |
DRH due to |
|
Base flow |
Ordinates of flood |
|
|
6-h UH |
1. cm ER |
1 cm ER |
|
|
hydrograph |
|
|
|
|
lagged by 6-h |
|
|
|
|
h |
m3/s |
m3/s |
m3/s |
m3/s |
m3/s |
m3/s |
|
0 |
0 |
0 |
|
0 |
10 |
10 |
|
6 |
20 |
20 |
0 |
20 |
10 |
30 |
|
12 |
60 |
60 |
20 |
80 |
10 |
90 |
|
18 |
150 |
150 |
60 |
210 |
10 |
220 |
|
24 |
120 |
120 |
150 |
270 |
10 |
280 |
|
30 |
90 |
90 |
120 |
210 |
10 |
220 |
|
36 |
66 |
66 |
90 |
156 |
10 |
166 |
|
42 |
50 |
50 |
66 |
116 |
10 |
126 |
|
48 |
32 |
32 |
50 |
82 |
10 |
92 |
|
54 |
20 |
20 |
32 |
52 |
10 |
62 |
|
60 |
10 |
10 |
20 |
30 |
10 |
40 |
|
66 |
0 |
0 |
10 |
10 |
10 |
20 |
|
72 |
|
|
0 |
0 |
10 |
10 |