The rocket equation

The Tsiolkovsky rocket equation, however, takes this into account. The motion of the rocket is governed by the conservation of momentum. When the rocket and internal gases are moving as one unit, the overall momentum, the product of mass and velocity, is equal to . Thus, for a total mass of rocket and gas moving at velocity

$mv = \left(m_r + m_g\right)v = P_1$

As the gases are expelled through the rear of the rocket, the overall momentum of the rocket and fuel has to remain constant as long as no external forces act on the system. Thus, if a very small amount of gas $\mathrm{d}m$ is expelled at velocity relative to the rocket (either in the direction of or in the opposite direction), the overall momentum of the system (sum of rocket and expelled gas) is

$\left(m - \mathrm{d}m\right) \left(v+\mathrm{d}v_r\right) + \mathrm{d}m \left(v + v_e\right) = P_2$

As has to equal to conserve momentum

$mv = \left(m - \mathrm{d}m\right) \left(v+\mathrm{d}v_r\right) + \mathrm{d}m \left(v + v_e\right)$

and by isolating the change in rocket velocity $\mathrm{d}v_r$

$\left(m-\mathrm{d}m\right) \mathrm{d}v_r = -v_e\mathrm{d}m$
$\therefore dv_r = -\frac{\mathrm{d}m}{\left(m-\mathrm{d}m\right)} v_e$

The negative sign in the equation above indicates that the rocket always changes velocity in the opposite direction of the expelled gas, as intuitively expected. So if the gas is expelled in the opposite direction of the rocket motion (so is negative), then the change in the rocket velocity will be positive and it will accelerate.

At any time the quantity $M = m-\mathrm{d}m$ is equal to the residual mass of the rocket (dry mass + propellant) and $\mathrm{d}m = \mathrm{d}M$ denotes it change. If we assume that the expelled velocity of the gas remains constant throughout, we can easily find the incremental change in velocity as the rocket changes from an initial mass to a final mass . So,

This equation is known as the Tsiolkovsky rocket equation and is applicable to any body that accelerates by expelling part of its mass at a specific velocity. Even though the expulsion velocity may not remain constant during a real rocket launch we can refer to an effective exhaust velocity that represent a mean value over the course of the flight.

The Tsiolkovsky rocket equation shows that the change in velocity attainable is a function of the exhaust jet velocity and the ratio of original take-off mass (structural weight + fuel = ) to its final mass (structural mass + residual fuel = ). If all of the propellant is burned, the mass ratio expresses how much of the total mass is structural mass, and therefore provides some insight into the efficiency of the rocket.

In a nutshell, the greater the ratio of fuel to structural mass, the more propellant is available to accelerate the rocket and therefore the greater the maximum velocity of the rocket.

So in the ideal case we want a bunch of highly reactant chemicals magically suspended above an ultralight means of combusting said fuel.

In reality this means we are looking for a rocket propelled by a fuel with high efficiency of turning chemical energy into kinetic energy, contained within a lightweight tankage structure and combusted by a lightweight rocket engine. But more on that later!

Thrust

Often, we are more interested in the thrust created by the rocket and its associated acceleration . By dividing the rocket equation above by a small time increment and again assuming to remain constant

$a_r = \frac{\mathrm{d}v_r}{\mathrm{d}t} = - \frac{\mathrm{d}M}{\mathrm{d}t} \frac{v_e}{M} = \frac{\dot{M}}{M} v_e$

and the associated thrust acting on the rocket is

$F_r = Ma_r = \dot{M} v_e$

where $\dot{M}$ is the mass flow rate of gas exiting the rocket. If the differences in exit pressure of the combustion gases and surrounding ambient pressure are accounted for this becomes:

$F_r = \dot{M} v_e + (p_e - p_{ambient}) A_e$

where is the jet velocity at the nozzle exit plane, is the flow area at the nozzle exit plane, i.e. the cross-sectional area of the flow where it separates from the nozzle, is the static pressure of the exhaust jet at the nozzle exit plane and $p_{ambient}$ the pressure of the surrounding atmosphere.

This equation provides some additional physical insight. The term $\dot{M} v_e$ is the momentum thrust which is constant for a given throttle setting. The difference in gas exit and ambient pressure multiplied by the nozzle area provides additional thrust known as pressure thrust. With increasing altitude the ambient pressure decreases, and as a result, the pressure thrust increases. So rockets actually perform better in space because the ambient pressure around the rocket is negligibly small. However, also decreases in space as the jet exhaust separates earlier from the nozzle due to overexpansion of the exhaust jet. For now it will suffice to say that pressure thrust typically increases by around 30% from launchpad to leaving the atmosphere, but we will return to physics behind this in the next post.

Impulse and specific impulse

The overall amount of thrust is typically not used as an indicator for rocket performance. Better indicators of an engine’s performance are the total and specific impulse figures. Ignoring any external forces (gravity, drag, etc.) the impulse is equal to the change in momentum of the rocket (mass times velocity) and is therefore a better metric to gauge how much mass the rocket can propel and to what maximum velocity. For a change in momentum $\Delta p$ the impulse is

$I = \Delta p = \Delta (mv) = \Delta(\frac{F}{a}v) = F_{average} \Delta t$

So to maximise the impulse imparted on the rocket we want to maximise the amount of thrust acting over the burn interval $\Delta t$ . If the burn period is broken into a number of finite increments, then the total impulse is given by

$I = \sum_{n=1}^{end} F_n \Delta t_n$

Therefore, impulse is additive and the total impulse of a multistage rocket is equal to the sum of the impulse imparted by each individual stage.

By specific impulse we mean the net impulse imparted by a unit mass of propellant. It’s the efficiency with which combustion of the propellant can be converted into impulse. The specific impulse is therefore a metric related to a specific propellant system (fuel + oxidiser) and essentially normalises the exhaust velocity by the acceleration of gravity that it needs to overcome:

$I_{sp} = v_e/g$

where is the effective exhaust velocity and =9.81. Different fuel and oxidiser combinations have different values of $I_{sp}$ and therefore different exhaust velocities.

A typical liquid hydrogen/liquid oxygen rocket will achieve an $I_{sp}$ around 450 s with exhaust velocities approaching 4500 m/s, whereas kerosene and liquid oxygen combinations are slightly less efficient with $I_{sp}$ around 350 s and around 3500 m/s. Of course, a propellant with higher values of $I_{sp}$ is more efficient as more thrust is produced per unit of propellant.

Delta-v and mass ratios

The Tsiolkovsky rocket equation can be used to calculate the theoretical upper limit in total velocity change, called delta-v, for a certain amount of propellant mass burn at a constant exhaust velocity . At an altitude of 200 km an object needs to travel at 7.8 km/s to inject into low earth orbit (LEO). If we start from rest, this means a delta-v equal to 7.8 km/s. Accounting for frictional losses and gravity, the actual requirement rocket scientists need to design for is just shy of delta-v=10 km/s. So assuming a lower bound effective exhaust velocity of 3500 m/s, we require a mass ratio of…

$\Delta v = \left|v_e\right| \ln \frac{M_0}{M_f} \Rightarrow \ln \frac{M_0}{M_f} = \frac{10000}{3500}=2.857$
$\therefore \frac{M_0}{M_f} = e^{2.86} = \underline{17.4}$

to reach LEO. This means that the original rocket on the launch pad is 17.4 times heavier than when all the rocket fuel is depleted!

Just to put this into perspective, this means that the mass of fuel inside the rocket is SIXTEEN times greater than the dry structural mass of tanks, payload, engine, guidance systems etc. That’s a lot of fuel!

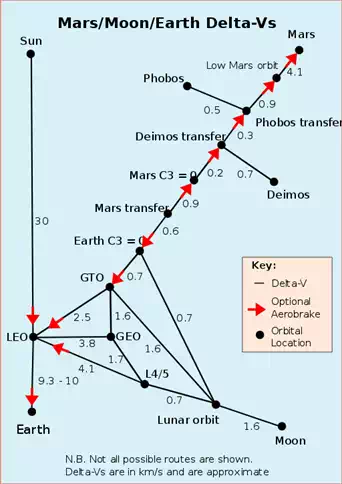
Delta-v figures required for rendezvous in the solar system. Note the delta-v to get to the Moon is approximately 10 + 4.1 + 0.7 + 1.6 = 16.4 km/s and thus requires a whopping mass ratio of 108.4 at an effective exhaust velocity of 3500 m/s.

The rocket’s initial mass to its final mass

$\frac{M_0}{M_f} = e^{\Delta v / v_e}$

is known as the mass ratio. In some cases, the reciprocal of the mass ratio is used to calculate the mass fraction:

$\text{Mass fraction} = 1 - \left(\frac{M_0}{M_f}\right)^{-1}$

The mass fraction is necessarily always smaller than 1, and in the above case is equal to $1 - 17.4^{-1} = 94.3$ .

So 94% of this rocket’s mass is fuel!

Such figures are by no means out of the ordinary. In fact, the Space Shuttle had a mass ratio in this ballpark (15.4 = 93.5% fuel) and Europe’s Ariane V rocket has a mass ratio of 39.9 (97.5% fuel).

If anything, flying a rocket means being perched precariously on top of a sea of highly explosive chemicals!

The reason for the incredibly high amount of fuel is the exponential term in the above equation. The good thing is that adding fuel means we have an exponential law working in our favour: For each extra gram of fuel we can pack into the rocket we get a superlinear (better than linear) increase in delta-v. On the downside, for every piece of extra equipment, e.g. payload, we stick into the rocket we get an equally exponential reduction in delta-v.

In reality, the situation is obviously more complex. The point of a rocket is to carry a certain payload into space and the distance we want to travel is governed by a specific amount of delta-v (see figure to the right). For example, getting to the Moon requires a delta-v of approximately 16.4 km/s which implies a whopping mass ratio of 108.4. Therefore, if we wish to increase the payload mass, we need to simultaneously increase propellant mass to keep the mass ratio at 108.4. However, increasing the amount of fuel increases the loads acting on the rocket, and therefore more structural mass is required to safely get the rocket to the Moon. Of course, increasing structural mass similarly increases our fuel requirement, and off we go on a nice feedback loop…

This simple example explains why the mass ratio is a key indicator of a rocket’s structural efficiency. The higher the mass ratio the greater the ratio of delta-v producing propellant to non-delta-v producing structural mass. All other factors being equal, this suggests that a high mass ratio rocket is more efficient because less structural mass is needed to carry a set amount of propellant.

The optimal rocket is therefore propelled by high specific impulse fuel mixture (for high exhaust velocity), with minimal structural requirements to contain the propellant and resist flight loads, and minimal requirements for additional auxiliary components such as guidance systems, attitude control, etc.

For this reason, early rocket stages typically use high-density propellants. The higher density means the propellants take up less space per unit mass. As a result, the tank structure holding the propellant is more compact as well. For example, the Saturn V rocket used the slightly lower specific impulse combination of kerosene and liquid oxygen for the first stage, and the higher specific impulse propellants liquid hydrogen and liquid oxygen for later stages.

Closely related to this, is the idea of staging. Once, a certain amount of fuel within the tanks has been used up, it is beneficial to shed the unnecessary structural mass that was previously used to contain the fuel but is no longer contributing to delta-v. In fact, for high delta-v missions, such as getting into orbit, the total dry-mass of the rockets we use today is too great to be able to accelerate to the desired delta-v. Hence, the idea of multi-stage rockets. We connect multiple rockets in stages, incrementally discarding those parts of the structural mass that are no longer needed, thereby increasing the mass ratio and delta-v capacity of the residual pieces of the rocket.

Cost

The cost of getting a rocket on to the launch pad can roughly be split into three components:

1. Propellant cost.

2. Cost of dry mass, i.e. rocket casing, engines and auxiliary units.

3. Operational and labour costs.

As we saw in the last section, more than 90% of a rocket take-off mass is propellant. However, the specific cost (cost per kg) of the propellants is multiple orders of magnitude smaller than the cost per unit mass of the rocket dry mass mass, i.e. the raw material costs and operational costs required to manufacture and test them. A typical propellant combination of kerosene and liquid oxygen costs around $2/kg, whereas the dry mass cost of an unmanned orbital vehicle is at least $10,000/kg. As a result, the propellant cost of flying into low earth orbit is basically negligible.

The incredibly high dry mass costs are not necessarily because the raw material, predominantly high-grade aerospace metals, are prohibitively expense, rather they cannot be bought at scale because of the limited number of rockets being manufactured. Second, the criticality of reducing structural mass for maximising delta-v means that very tight safety factors are employed. Operating a tight safety factor design philosophy while ensuring sufficient safety and reliability standards under the extreme load conditions exerted on the rocket means that manufacturing standards and quality control measures are by necessity state-of-the-art. Such procedures are often highly specialised technologies that significantly drive up costs.

To clear these economic hurdles, some have proposed to manufacture simple expendable rockets at scale, while others are focusing on reusable rockets. The former approach will likely only work for unmanned smaller rockets and is being pursued by companies such as Rocket Lab Ltd. The Space Shuttle was an attempt at the latter approach that did not live up to its potential. The servicing costs associated with the reusable heat shield were unexpectedly high and ultimately forced the retirement of the Shuttle. Most, recently Elon Musk and SpaceX have picked up the ball and have successfully designed a fully reusable first stage.