What Creates Lift – How Do Wings Work?

How airplanes fly is one one of the most fundamental questions in aerospace engineering. Given its importance to flight, it is surprising how many different and oftentimes wrong explanations are being perpetuated online and in textbooks. Just throughout my time in school and university, I have been confronted with several different explanations of how wings create lift.

Most importantly, the equal transit time theory, explained further below, are taught in many school textbooks and therefore instil faulty intuitions about lift very early on. This is not necessarily because more advanced theories are harder to understand or require a lot maths. In fact, the theory that requires the simplest assumptions and least abstraction is typically considered to be the most useful.

In science, the simplicity of a theory is a hallmark of its elegance. According to Einstein (or Louis Zukofsky or Roger Sessions or William of Ockham…I give up, who knows), “everything should be made as simple as possible, but not simpler.” Hence, the strength of a theory is related to:

● The simplicity of its assumptions, ideally as few as possible.

● The diversity of phenomena the theory can explain, including phenomena that other theories could not explain.

Keeping this definition in mind, let’s investigate some popular theories about how aircraft create lift.

The first explanation of lift that I came across as a middle school student was the theory of “Equal Transit Times”. This theory assumes that the individual packets of air flowing across the top and bottom surfaces must reach the trailing edge of the airfoil at the same time. For this to occur, the airflow over the longer top surface must be travelling faster than the air flowing over the bottom surface. Bernoulli’s principle, i.e. along a streamline an increasing pressure gradient causes the flow speed to decrease and vice versa, is then invoked to deduce that the speed differential creates a pressure differential between the top and bottom surfaces, which invariable pushes the wing up. This explanation has a number of fallacies:

● There is no physical law that requires equal transit times, i.e. the underlying assumptions are certainly not as simple as possible.

● It fails to explain why aircraft can fly upside down, i.e. does not explain all phenomena.

As this video shows, the air over the top surface does indeed flow faster than on the bottom surface, but the flows certainly do not reach the trailing edge at the same time. Hence, this theory of equal transit times is often referred to as the “Equal Transit Time Fallacy”.

In order to generalise the above theory, while maintaining the mathematical relationship between speed and pressure given by Bernoulli’s principle, we can relax the initial assumption of equal transit time. If we start from a phenomenological observation of streamlines around anairfoil, as depicted schematically below, we see can see that the streamlines are bunched together towards the top surface of the leading edge, and pinched together towards the bottom surface of the leading edge. The flow between two adjacent streamlines is often called astreamtube, and the upper and lower streamtubes are highlighted in shades of blue in the figure below. The definition of a streamline is the line a fluid particle would traverse as it flows through space, and thus, by definition, fluid can never cross a streamline. As two adjacent streamlines form the boundaries of the streamtubes, the mass flow rate through each streamtube must be conserved, i.e. no fluid enters from the outside, and no fluid particles are created or destroyed. To conserve the mass flow rate in the upper streamline as it becomes narrower, the fluid must flow faster. Similarly, to conserve the mass flow rate in the lower streamtube as it widens, the fluid must slow down. Hence, in accordance with the speed-pressure relationship of Bernoulli’s principle, this constriction of the streamtubes means that we have a net pressure differential that generates a lift force.

Flow lines around a NACA 0012 airfoil at 11° angle of attack, with upper and lower streamtubes identified.

Of course, this theory does not explain why the upper streamtube contracts and the lower streamtube expands in the first place. An intuitive explanation for this involves the argument that the angle of attack obstructs the flow more towards the bottom of the airfoil than towards the top. However, this does not explain how asymmetric airfoils with pronounced positive camber at zero angle of attack, as shown in the figure below, create lift. In fact, such profiles were successfully used on early aircraft due to their resemblance to bird wings. Again, this theory does not explain all the physical phenomena we would like it to explain, and is therefore not the rigorous theory we are looking for.

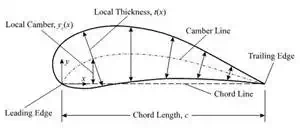
Asymmetric airfoil with pronounced camber [1]

One of the explanations for lift, that was relayed to me as an undergraduate by a university professor, is the simple concept of Newton’s third law. Aerodynamicists like to draw control volumes around bodies, and then compare the properties of individual streamlines as they enter and leave the control volume. If we apply such a control volume around a typical airfoil with a positive angle of attack, such as the one two figures above, we see that the airfoil redirects the flow from initially horizontal to pointing downwards. Therefore, the wing has exerted a net downwards force on the airflow and by equal and opposite reaction, the air must exert an upwards force on the airfoil, hence producing lift.

One way we can invalidate a theory is by providing a counterexample. For example, consider an airfoil at zero angle of attack with a pronounced upwards camber, i.e. an asymmetric airfoil profile, as shown in the figure below.

High camber airfoil with control volume

In this case, the air entering and leaving the control volume is flowing in the same direction and hence there is no net downwards force on the air. Nevertheless, this cambered wing does generate good amounts of lift and the benefits of cambered airfoils in terms of increasing the lift coefficient and increasing stall speeds have been known since the early 1800’s. Hence, this action-reaction theory of lift does not account for the diversity of phenomena we would like explain with our theory of lift.

We can now appreciate that the approaches based purely on Bernoulli’s principle, or the downwards redirection of fluid, are either based on faulty assumptions or do not explain all physical phenomena we observe in experiments. What we do know from fundamental balance of forces, in order for a net upwards lift to act on an airfoil, the force exerted on the upper surface by the surrounding fluid must be less than the force exerted on the the lower surface. As pressure is always greater than zero, the fluid flowing over the top surface of the airfoil must press down less than the fluid underneath the airfoil is pressing up. Assuming that the surface areas of the top and bottom surfaces are of similar size, this condition requires that the local fluid pressure is lower on the top surface than on the bottom surface.

So the question remains, what causes this pressure difference?

To answer this question we will refer to the journal paper by Dr Holger Babinsky, which is free to download. If we consider purely stead-state flow and neglect the effects of gravity, surface tension and friction we can derive some very basic, yet insightful, equations that explain the aforementioned pressure difference. Intuitively, this argument shows that a force acting parallel to a streamline causes the flow to accelerate or decelerate along its tangential path. Conversely, a force acting perpendicular to the flow direction causes the streamline to curve.

The first case is described mathematically by Bernoulli’s principle and depicted in the figure below. If we imagine a small fluid particle of finite length l situated in a field of varying pressure, then the front and back surfaces of the particle will experience different pressures. Say the pressure increases along the streamline, then the force acting on the front face pointing in the direction of motion is greater than the force acting on the rear surface. Hence, according to Newton’s second law, this increasing pressure field along the streamline causes the flow speed to decrease and vice versa. However, this approach is valid only along a single streamline. Bernoulli’s principle can not be used to relate the speed and pressures of adjacent streamlines. Thus, we can not use Bernoulli’s principle to compare the flows on the bottom and top surfaces of an airfoil, and therefore can say little about their relative pressures and speeds.

Flow along a straight streamline [2]

However, consider the curved streamlines shown in the figure below. If we assume that the speed of the particle travelling along the curved streamline is constant, then Bernoulli’s principle states that the pressure along the streamline can not change either. However, the velocity vector v is changing, as the direction of travel is changing along the streamline. According to Newton’s second law, this change in velocity, i.e. acceleration, must be caused by a net centripetal force acting perpendicular to the direction of the flow. This net centripetal force must be caused by a pressure differential on either side of the particle as we have ignored the influence of gravity and friction. Hence, a curved streamline implies a pressure differential across it, with the pressure decreasing towards the centre of curvature.

Flow along a curved streamline [2]

Mathematically, the pressure difference across a streamline in the direction n pointing outwards from the centre of curvature is

where R is the radius of curvature of the flow and  is the density of the fluid.

One positive characteristic of this theory is that it explains other phenomena outside our interest in airfoils. Vortices, such as tornados, consist of concentric circles of streamlines, which suggests that the pressure decreases as we move from the outside to the core of the vortex. This observation agrees with our intuitive understanding of tornados sucking objects into the sky.

With this understanding we can now return to the study of airfoils. Consider the simple flow path along a curved plate shown in the figure below. At point A the flow field is unperturbed by the presence of the airflow and the local pressure is equal to the atmospheric pressure . As we move down along the dashed curve we see that the flow starts to curve around the curved plate. Hence, the pressure is decreasing as we move closer to the airfoil surface and . On the bottom half the situation is reversed. Point C is again undisturbed by the airflow but the flow is increasingly curved as me closer to D. However, when moving from C to D, the pressure is increasing because pressure increases moving away from the centre of curvature, which on the bottom of the airfoil is towards point C. Thus,  and by the transitive property  such that the airfoil experiences a net upward lift force.

Flow around a curved airfoil [2]

From this exposition we learn that any shape that creates curvature in the flow field can generate lift. Even though friction has been neglected in this analysis, it is crucial in forcing the fluid to adhere to the surfaces of the airfoil via a viscous boundary layer. Therefore, the inclusion of friction does not change the theory of lift due to streamline curvature, but provides an explanation for why the streamlines are curved in the first place.

A couple of interesting observations follow from the above discussion. Nature typically uses thin wings with high camber, whereas man-made flying machines typically have thicker airfoils due to their improved structural performance, i.e. stiffness. In the figure below, the deep camber thinner wing shows highly curved flow on both the top and bottom surfaces.

Deep camber thin wing with high lift [2]

Shallow camber thick wing with less lift [2]

The more shallow camber thicker wing has flow curved in two different directions on the bottom surface and will therefore result in less pressure difference between the top and bottom surfaces. Thus, for maximum lift, the thin, deeply cambered airfoils used by birds are the optimum configuration.

In conclusion, we have investigated a number of different theories how lift is created around airfoils. Each theory was investigated in terms of the simplicity and validity of its underlying assumptions, and the diversity of phenomena it can describe. The theories based on Bernoulli’s principle, such as the equal transit time theory and the contraction of streamtubes theory, were either based on faulty initial assumptions, i.e. equal time, or failed to explain why streamtubes should contract or expand in the first place. The theory based on Newton’s third law, that the airflow deflects airflow down, and as a reaction a lift force is generated, was shown to be lacking, as it did not account for thick cambered wings at zero angle of attack.

The most robust theory is that curved bodies induce curved streamlines, as the inherent viscosity of the fluid forces the fluid to adhere to the surface of the body via a boundary layer. The centripetal forces that arise in the curved flow lead to a drop in pressure across the streamlines towards the centre of curvature. The elegance of this theory is that it not only explains the phenomena of lift in airfoils, but also other phenomena involving curved flow.